Case Studies and Project Ideas: Malaria

Disease Background:

Malaria is a major cause of illness and death in many tropical and subtropical countries. It results from infection by a species of protozoan parasite of the genus Plasmodium. The word malaria comes from the Italian, and literally means "bad air", since malaria was believed to result from exposure to marsh air. By the end of the nineteenth century, however, scientists recognized that malaria is passed from one human host to the next by the bite of an infected female mosquito of the genus Anopheles.

In the western hemisphere, malaria is believed to have not been present until sometime after Columbus' arrival. In the "old world", however, the presence of malaria appears to be much more ancient. Hippocrates, in Greece during the fourth century, B.C., described a series of fevers and illnesses that appear very similar to malaria. The disease was also present in Italy at that time, and centuries later may have contributed to much of the decline of the Roman Empire.

Early attempts to control malaria focused on the symptoms of the disease itself, rather than the underlying cause. In the seventeenth century, the bark from a Peruvian tree was discovered to provide relief from certain types of fevers. This also allowed scientists to begin to define malaria as separate from other fever-causing illnesses, based on whether the tree bark had any effect in treating the patient's fever.

It was not until the twentieth century, however, that effective means of treatment were found. Once the specific species of mosquito that carries malaria was discovered, synthetic antimalarials were developed, and could be used for both treatment and prevention. Pesticides were also used to eradicate the disease-carrying insects, but these have only met with limited success, as the mosquitoes tend to soon develop immunities.

Epidemiology

While the transmission of many diseases (such as AIDS) is from one host to the next , this is not the case for malaria. Instead, diseases like malaria also involve an intermediate host, called a vector . The parasite responsible for malaria must spend part of its life-cycle in the vector, and so the normal path of transmission is from an infected human to a vector, and then from the vector back to another human host.

The first attempts to develop a quantitative understanding of malaria transmission were carried out by Ross in the early twentieth century. These early studies, and the subsequent work by the epidemiologist George Macdonald in the 1950's, provided an important framework for all future studies of malarial infection.

Since the spread of malaria depends on two population groups (humans and insects), a successful model of the disease must examine these groups individually as well as their interactions. In the human population, new infections are acquired at a rate that depends on the number of mosquito bites per person per unit time, the probability that a biting mosquito is infected, that a bitten human is uninfected, and on the chance that an uninfected person will actually develop an infection once bitten. Some infected people will also recover, and thus return to the pool of uninfected humans.

As for the mosquito population, the proportion infected depends on the number of bites per mosquito per unit time, on the probability the biting mosquito is uninfected and that the bitten human is infected, and on the chance that an uninfected mosquito acquires infection from biting an infectious person. The loss of infected mosquitoes arises from their death, as they do not appear to recover from malarial infection.

Sudan: A Case Study

Sudan, a country in eastern Africa, presents an interesting study for malaria epidemics. It is a developing country located primarily between Egypt, Chad, Ethiopia and the Red Sea. The capital of the nation is Khartoum with a population of around half a million. In the 1950s, Sudan overall had approximately 8 million people, but the country now has over 20 million. This can be attributed largely to the fact that during the 1950s, Sudan had a extremely high yearly birth rate of about 50 births per 1000 people as well as a large refugee population entering from other countries. The population has exploded despite Sudan having a quite high yearly death rate of 20 deaths per 1000 people. Much of the country is remote and medical facilities are in scant supply. Thus dealing with epidemics such as malaria is a very difficult challenge to the leaders of this country. Diseases in the area typically sweep from east to west, from Chad to the Red Sea, along a corridor known as the "epidemic belt" of the Sudan.

Soon after Ross demonstrated the cycle of the malaria parasite, Andrew Blafour was appointed to the post of medical officer of health in Sudan. He undertook a major study of the distribution of mosquitoes and set out on a "mosquito brigade" to eradicate mosquitoes and malaria. Although these efforts were somewhat effective, the spread of malaria continued in Sudan for many decades.

During the late 1940's into 1950, the number of cases of malaria increased, due in part to the Second World War and its aftermath, leading to a decline in malaria control measures. In 1950, 351,901 new cases of malaria were reported in Sudan, representing approximately 8% of all diseases reported at medical institutions. The annual incidence of malaria per 100,000 people ranged from approximately 3,000 to 4,000 between 1950 and 1955.

Although malaria is a direct or indirect cause of death in a large proportion of the population of the Sudan, it is rarely reported as a cause since it is often confused with other diseases, especially at rural hospitals. Thus, during the 1940's, the average mortality rate due to malaria was 2.0 per 100,000, while from 1950 to 1959 the rate was 3.1 per 100,000. The fact that this period also was a time of expansion for the health service may explain part of this increase, as physicians became more likely to report malaria on the death certificates.

Building the Model:

Using STELLA, it is possible to make a model of malaria that is quite accurate. This model looks at four populations:

Healthy villagers
Sick villagers
Healthy mosquitoes
Infected mosquitoes

In this model we do not assume any constant populations; in other words, people are being born and people are dying (both of the disease and from "natural" causes). The same is true for mosquitoes.

The algorithms for this model are shown below:

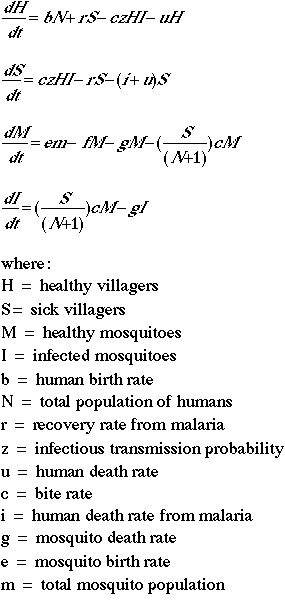
Use these values for your initial run:

Heathy villagers = 100
Sick villagers = 0
Healthy mosquitoes = 1000
Infected mosquitoes = 10
Human birth rate = 5%
Bite rate = 60%
Human death rate = 2%
Human death rate from malaria = 50%
Mosquito birth rate = 20.2%
Mosquito death rate = 20%
Recovery rate from malaria = 25%
Infectious transmission probability = 90%

Run this model for at least 100 years.

Some experiments to consider:

Investigate some ways to eradicate the disease. What parameters migh you change (and by how much) to eradicate the disease over a period of 50-100 years?
On the Web, you might wish to look at: http://www.brown.edu/Courses/Bio_160/Projects1999/malaria/malmain.html for an in-depth look at the epidemiology of malaria.
How might you add modeling components that look at the use of pesticides and their effect on the malaria epidemic. Suppose you have this effect:
Pesticides increase the mosquito death rate in the early stages of their application but the effect of pesticides decreases exponentially over
time.
How might you model this?
Developed by
The Shodor Education Foundation, Inc.
Copyright © 2000-2003
Questions or comments about this page should be directed to biomed@shodor.org