|
In this model, McGuffie and Henderson-Sellers present a relatively simple one-dimensional EBM, in which each latitude zone is evaluated independently. The fundamental equation:
states the energy balance: solar radiation coming in (Si) times the fraction reflected due to albedo (which is itself dependent on temperature) "balances out" the outgoing radiation (temperature-dependent) plus a transport out, or energy loss by a latitude zone to its colder neighbors.
The surface albedo, or the amount of incident radiation reflected back to the atmosphere, is, like all of the parameters in the energy balance algorithm, dependent on the surface temperature for a given zone or latitude. In this case, we have simply made the albedo a "toggle" parameter, alternating between two choices if the zonal temperature is more or less than the critical temperature. The critical temperature at which a zone "ices over", or glaciates:
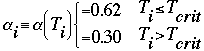
Radiation leaving the zone is calculated by:
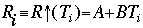
where A and B are longwave radiation loss constants which approximate the greenhouse effects of clouds, water vapor, and carbon monoxide.
Energy transport out of the system is a function of a transport constant, K, times the difference between the zonal temperature and the mean global temperature:
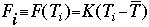
Zonal temperature can be calculated by rearrangment of several of the preceeding equations:
Note that additional fundamental equations were presented in the application page.
| 
The Shodor
Education Foundation, Inc.