|
Student: I am having some trouble deciding whether some of the more complex graphs are functions or not. Mentor: There are many ways to tell if it is a function. Student: Are there any that can be done by just looking at the graph? Mentor: As a matter of fact there is. It is called the vertical line test. Student: What does a vertical line have to do with functions? Mentor: Look at one of the graphs you have a question about. Then take a vertical line and place it on the graph. If the graph is a function, then no matter where on the graph you place the vertical line, the graph should only cross the vertical line once. Student: Really? Does that work all of the time? Mentor: Yes, all of the time. Let's try one. 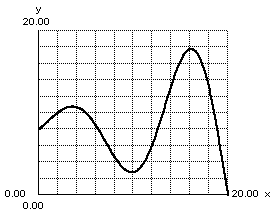 Can you tell me if that is a function in that plot? Student: Yes, that is. I can't put a vertical line anywhere on this graph and have it touch the function more than once. This works great, especially if I can see the grid. Mentor: What about common shapes like circles and squares? Student: They are not functions. Mentor: Can you tell me why? Student: They would not pass the vertical line test. Mentor: Why don't they pass? Student: Both of those shapes pass through the vertical line twice in lots of places! Look, here's just one example: 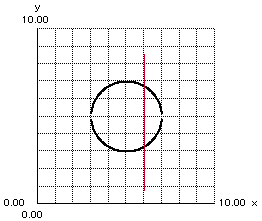 Mentor: Yes! Remember that hitting the vertical line more than once would mean that there are more than one y values for certain x values. This works for formulas when you are not sure whether they are functions. Any time you get more than one y value when you fill in an x value, it means that the formula is not a function. Having at most one y value for every x value means that there is a well-defined relationship, or in other words, a function. Student: That means that graphs such as x = 2 are not functions but graphs such as y = 2 are. Mentor: You are correct. Now lets try a harder one. 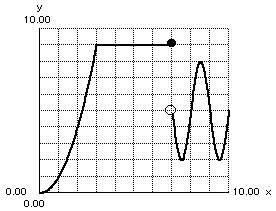 Student: What is going on at x=7? It looks like the graph jumps down? Mentor: That's right. It is up on the flat part at x=7, but for x larger than 7 it is on the curvy part. That colored in dot tells us that at 7 we should use the y-value 9, the dot with no shading tells us that the curvy part of the graph is what to use for anything larger than x = 7, but not for 7 itself. We call this a piecewise graph, and when we have this situation, we need to be told where the open and closed (filled or not) circles are. We call this a piecewise graph, and when we have this situation, we need to be told where the open and closed (filled or not) circles are. Student: If that is how you read it, then this is a function. Nowhere on this graph can I make a vertical line touch the function in more than one place. This makes things a lot easier and it makes sense. Mentor: Can you draw me a graph that is not a function? Student: Sure! Here's one: 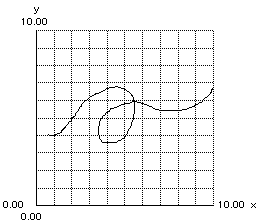 What are these called? Mentor: Well, every graph that describes a relationship between x and y is called a relation. Also, graphs that are drawn on the cartesian plane are called plane curves, 2-dimensional curves, or simply curves. So, functions are all of these things, but to answer your question, graphs that are not functions can be called by any of these names.

Please direct questions and comments about this project to Addison-Wesley math@aw.com © Copyright 1997-2001 The Shodor Education Foundation, Inc. © Copyright 2001 Addison-Wesley. All rights reserved. |