Mentor: We all know that we see our "reflection" in a mirror. If I draw a triangle on the board and this "mirror," what would the reflection look like?

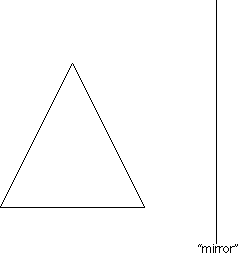Student 1: Well, I think that it would look just the same on the other side of the line. Like this:

Mentor: If I make an "X" on this line on the original triangle, where would it be on the reflected triangle?

Student 2: Well, when we reflected the triangle, we sort of flipped it over that "mirror," or the line, so it would be on the opposite side of the triangle, like this:

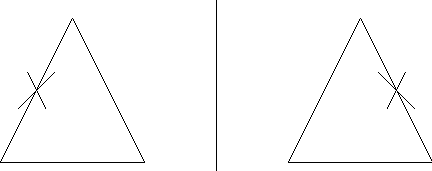Mentor: Great! We call that line the line of symmetry. See, the picture is symmetrical about the line.

Now that we know what it means to reflect an object, what do you think it means to rotate an object?

Student 3: I think it means that we just turn it. Kind of like spinning it around while the center stays in one place.

Mentor: That's good. Can you draw what this triangle would look like if we rotated it 90 degrees? Don't forget to draw the "x" on the right line!

Student 3: I think it would look like this:

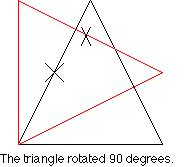Mentor: Very nice. Now we have one more transformation that we can do. Who can guess what it means to translate an object?

Student 4: Is that like moving it over?

Mentor: Good! A translation is like sliding the object over a certain distance. If I translated this triangle about 14 cm, who could draw what it would look like?

Student 4: I think it would look like this:

Mentor: Very nicely done! Thank you for including the "x" on the appropriate side of the triangle.

Please direct questions and comments about this project to Addison-Wesley math@aw.com
© Copyright 1997-2001 The Shodor Education Foundation, Inc.
© Copyright 2001 Addison-Wesley. All rights reserved.